Normal Distribution

For an   N(μ,σ²) distributed random variable X with given expected value μ and variance  σ², You can calculate

 

- density function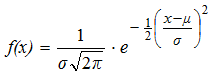    

- and distribution function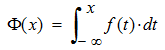

The graph of the density function ƒ(x) is often called a Gaussian or bell shaped curve. The distribution function  Φ(x) is called the Gaussian error function, because, according to Gauss, this distribution is assumed for the random errors in astronomical observations.

The expected value  μ  and variance  σ² are entered. For  μ=0  and  σ=1  you receive the standardized normal distribution.

Example:

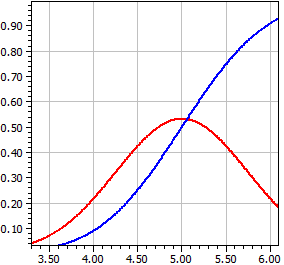
  μ = 5 ,      σ = .75

         x                     ƒ(x)                   Φ(x)      
  —————       –—————     —————–
  2                       0,00017844      0,00003167   
  2,33333333      0,00095649      0,00018859
  2,66666666      0,00420802      0,00093192
  2,99999999      0,01519465      0,00383038
  3,33333332      0,04503153      0,01313415
  3,66666665      0,10953585      0,03772017
  3,99999998      0,21868009      0,09121120
  4,33333331      0,35832381      0,18703139
  4,66666664      0,48189843      0,32836063
  4,99999997      0,53192304      0,49999998
  5,3333333        0,48189845      0,67163934
  5,66666663      0,35832383      0,81296859
  5,99999996      0,21868012      0,90878878
  6,33333329      0,10953586      0,96227982

See also:

Setting the graphics
Wikipedia: Normal distribution