Triangles by three Elements

Triangles are determined by three exterior magnitudes (sides or angles) if one of the following congruencies are complied.:

• sss     three sides are given.
• sww   one side and two angles are given.
• sws    two sides and the enclosed angle are given.
• Ssw    two sides and the angle opposite of the greater side are given.

Enter three exterior magnitudes (sides or angles), then the program computes the sides, the angles, the altitudes, the medians and the bisectors of the angles, the circumference and the area as well as the centers and radiuses of the inscribed and the circumscribed circle of the triangle.

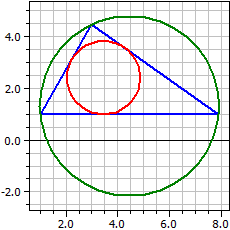
In addition the program draws the triangle with its inscribed and circumscribed circles.

If you enter two sides and the angle opposite of the shorter side, you get two solutions if they exist.

Example:

Given:  a=6, b=4 and α=60°

   Vertices : A(1|1)         B(7,899|1)     C(3|4,4641)    
      Sides : 6                  4                    6,89898        
    Angles : 60°               35,2644°       84,7356°       
 Altitudes : 3,98313       5,97469         3,4641         
 Medians : 4,77472       6,148             3,75513        
   Bisectr. : 4,38551       6,11664         3,5464         

Circumcir.: M(4,44949|1,31784)       ru = 3,4641
    Incircle : O(3,44949|2,41421)       r i = 1,41421

       Area : A = 11,9494   Perimeter : u = 16,899

See also:

Setting the graphics